Try the Free Math Solver or Scroll down to Tutorials!

Enter expression, e.g. (x^2-y^2)/(x-y)

Enter expression, e.g. x^2+5x+6

Enter expression, e.g. (x+1)^3

Enter a set of expressions, e.g. ab^2,a^2b

Enter equation to solve, e.g. 2x+3=4

Enter equation to graph, e.g. y=3x^2-1

Depdendent Variable

Number of equations to solve:

Equ. #1:

Equ. #2:

Equ. #3:

Equ. #4:

Equ. #5:

Equ. #6:

Equ. #7:

Equ. #8:

Equ. #9:

Solve for:

Enter inequality to solve, e.g. 2x+3>4

Enter inequality to graph, e.g. y<3x^2-1

Dependent Variable

Number of inequalities to solve:

Ineq. #1:

Ineq. #2:

Ineq. #3:

Ineq. #4:

Ineq. #5:

Ineq. #6:

Ineq. #7:

Ineq. #8:

Ineq. #9:

Solve for:

Math solver on your site

Please use this form if you would like
to have this math solver on your website,
free of charge.

Name:

Email:

Your Website:

Msg:

Rational Exponents and Radicals Definitions

Defintion 1: If x ≠ 0, then and is undefined

Defintion 2: If x ≠ 0, then 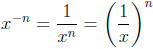for any rational number n
For any positive integer n and m

Defintion 3: If n is odd, then “nth root of a” is 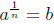such that
If n is even and a > 0, then “principal nth root of a” is 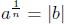such that

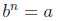
Defintion 4: If is real, then

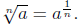
So, for odd n: 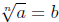such that 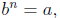for even n and a > 0: 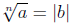such that
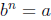
So, for odd n: 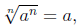for even n: 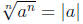for any a.

is	not a real number	if n is even and a < 0
	a rational number	if a is a perfect nth power (e.g. 9 = 3² is a perfect square)
	an irrational number	if a is not a perfect nth power

Defintion 5: If 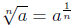is real, then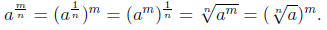

Defintion 6: If 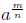is real, then

From now on r and s are any rational numbers, m, n and k are any intergers.

Rules

Rule 1:

Rule 2:

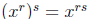
Rule 3:

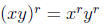
Rule 4: For

Rule 5: For

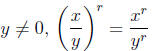
If 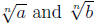are real then Rule 6: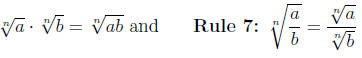

If all indicated roots are real then

Rule 8: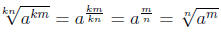

Sumplifying Radicals - Rationalizing denominator (square roots only!)

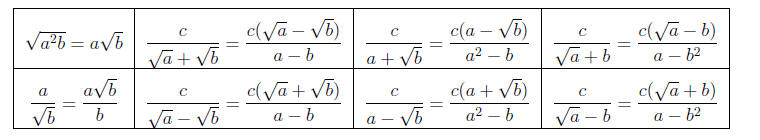
Simplifying Radicals - We will simplify in each step as example.

Step 1: Rewrite the expression with fractional exponents/powers.

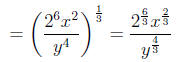By Rules 2, 3 and 5

Step 2: Reduce fractional powers

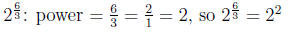
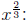power = 2/3 - already reduced

power = 4/3 - already reduced

Step 3: If fractional power > 1, write it as a mixed fraction, follow example steps.

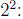power = 2 - not fractional power

power = 2/3 < 1

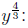power =

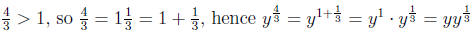
Step 4: Put things back in the main problem

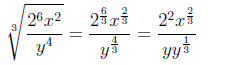
Step 5: Pull out factors with integer powers

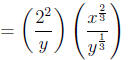
Step 6: Rationalize the denomenator of the 2nd factor

Denomenator 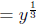, we need to make it , so multiply numerator by

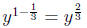
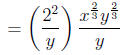[We get 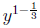by solving 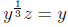for z]

Step 7: Pull out the common denomenator of the fractional power as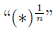

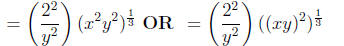
Step 8: Switch back to radical notation

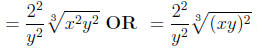—– Now it is fully simplified!