Irrational Numbers in General and Square Roots in
Particular
In Set 2 you learned that every rational number has a
decimal expansion which either
terminates or repeats. Thus if there exist any decimal expansions which do not
terminate and do not repeat, the numbers represented by these decimal expansions
cannot be rational.
1. Consider for instance the following number:
r = :123456789101112131415161718192021222324252627 ...
What is the pattern for the digits of this number? What will the next six digits
be?
Since this number does not have a repeating pattern, it is not rational. In
other
words, if m and n are any integers, then
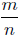 is never exactly equal to r . (However
is never exactly equal to r . (However
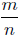 might be extremely close to r . For instance
might be extremely close to r . For instance
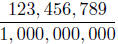 is very close
is very close
to r. (why?))
Numbers which are not rational - such as r - are called irrational numbers.
You might wonder why anybody would need irrational numbers. Indeed, rational
numbers are good enough to do a whole lot with. Since the rational numbers are
closed under addition, subtraction, multiplication, and division (except for
division
by 0), each of the following equations has a solution which is a rational
number:
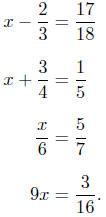
Verify the above statement by solving all of the equations
given and noticing that the
solutions are rational numbers.
Not all equations involving integers have solutions which are rational numbers,
however. In this set we will show that if N is an integer which is not a
\perfect
square" (such as 9, 16, 25, 36, 49, or 64) then the solution to the equation x2
= N
is not a rational number. You are going to see that if a and b are any integers
then
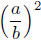 is never exactly equal to N , so that
is never exactly equal to N , so that
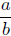 is never exactly equal to
is never exactly equal to
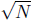 .
.
(Actually, if N > 0 then the equation x2
= N has two solutions: 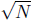 and −
and −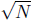 .
.
What we are going to show is the 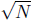 is always
either an integer { in which case N
is always
either an integer { in which case N
is a perfect square { or an irrational number.)
As preparation for the proof, we will notice something about squaring fractions
which
are in lowest terms.
2. Using the fact that

find the prime factorizations for 1002, 912, 2472
, 6672, and 10,1272
.
Based on these examples, state a general rule for the prime factorization of the
square
of a number.
Which of the following fractions are in lowest terms?

If a and b are integers, which of the following
possibilities can occur?
(1) 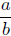 and
and
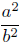 are both in lowest terms.
are both in lowest terms.
(2) 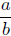 is in lowest
terms but
is in lowest
terms but 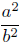 is not.
is not.
(3) 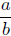 is not in lowest
terms but
is not in lowest
terms but 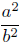 is in lowest terms.
is in lowest terms.
(4) 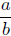 and
and
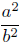 are both not in lowest terms.
are both not in lowest terms.
Explain why!
3. We want to show that if a and b are integers such that
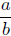 is not an integer and
is not an integer and
if furthermore N is an integer, then 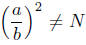 . To
start with. let's think about
. To
start with. let's think about
when a fraction can be an integer.
Which of the following rational numbers are integers?

Which of these fractions is in lowest terms?
If a and b are integers, what has to be true in order for
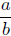 to be in lowest terms
and
to be in lowest terms
and
also to be an integer?
If a and b are integers, when is it possible for
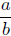 to be in lowest terms
and for
to be in lowest terms
and for 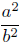 to
to
be an integer?
If 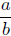 is a fraction in
lowest terms, which of the following are possible values
is a fraction in
lowest terms, which of the following are possible values
for 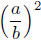 ?
?
How does your answer change if
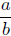 is not in lowest
terms?
is not in lowest
terms?
4. If N is an integer and a and b are integers and
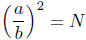 , explain why
, explain why
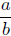
has to be an integer. Explain why N has to be a perfect square.
Explain why if N is not a perfect square then
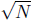 has to be irrational.
has to be irrational.
Which of the numbers listed above in  have
irrational square roots?
have
irrational square roots?