Exponents and Radicals
Integer Exponents
Repeated multiplication can be written in exponential
form.
| Repeated Multiplication |
Exponential Form |
 |
 |
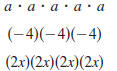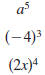In general, if a is a real number, variable, or algebraic
expression and n is a
positive integer, then
where n is the exponent and a is the base. The expression
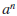is read “a to the nth
power.” An exponent can be negative as well. Property 3 below shows how to use
a negative exponent.
| Properties of Exponents
Let a and b be real numbers, variables, or algebraic
expressions, and let m
and n be integers. (All denominators and bases are nonzero.)
| Property |
Example |
 |
 |
|
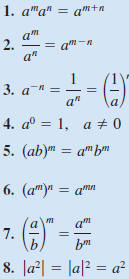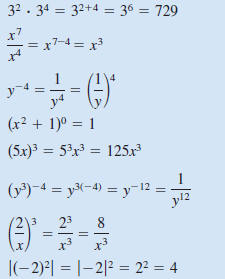It is important to recognize the difference between
expressions such as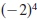 
and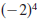 
. In 
,
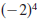the parentheses indicate that the exponent applies to the
negative sign as well as to the 2, but in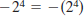 
the exponent applies only
to the 2. So,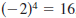 
whereas
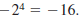It is also important to know
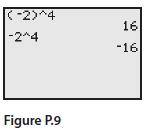
when to use parentheses when evaluating exponential expressions using a
graphing calculator. Figure P.9 shows that a graphing calculator follows the
order
of operations.
The properties of exponents listed on the preceding page
apply to all integers
m and n, not just positive integers. For instance, by Property 2, you can write
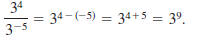
Example 1 Using Properties of Exponents
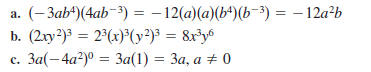
Now try Exercise 15.
Example 2 Rewriting with Positive Exponents
 |
Property 3 |
 |
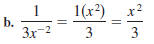The exponent -2 does not apply to 3. |
 |
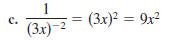The exponent -2 does apply to 3. |
 |
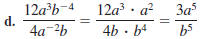Properties 3 and 1 |
 |
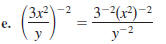Properties 5 and 7 |
 |
Property 6 |
 |
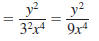Property 3, and simplify. |
| STUDY TIP
Rarely in algebra is there only
one way to solve a problem.
Don’t be concerned if the steps
you use to solve a problem are
not exactly the same as the steps
presented in this text. The
important thing is to use steps
that you understand and, of
course, that are justified by the
rules of algebra. For instance,
you might prefer the following
steps for Example 2(e).
|
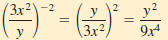
Now try Exercise 19.
Example 3 Calculators and Exponents
| Expression |
Graphing Calculator Keystrokes |
Display |
 |
 |
 |
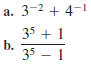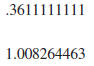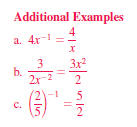
Now try Exercise 23.
TECHNOLOGY TIP
The graphing calculator keystrokes given in this text
may not be the same as the keystrokes for your graphing calculator. Be sure
you are familiar with the use of the keys on your own calculator.
Scientific Notation
Exponents provide an efficient way of writing and
computing with very large (or
very small) numbers. For instance, there are about 359 billion billion gallons
of
water on Earth—that is, 359 followed by 18 zeros.
359,000,000,000,000,000,000
It is convenient to write such numbers in scientific
notation. This notation has
the form 
and n is an integer. So, the number
of
gallons of water on Earth can be written in scientific notation as
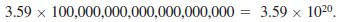
The positive exponent 20 indicates that the number is
large (10 or more) and
that the decimal point has been moved 20 places. A negative exponent indicates
that the number is small (less than 1). For instance, the mass (in grams) of one
electron is approximately
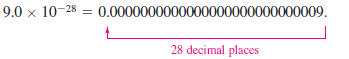
Example 4 Scientific Notation
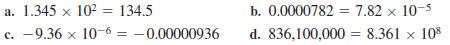
Now try Exercise 31.
Activities
1. Simplify:
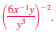
Answer:
2. Write in scientific notation: 39,000,000.
Answer: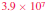
3. Write in decimal notation: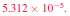 
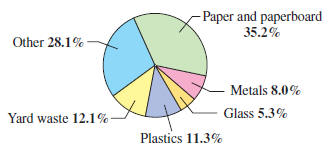
Answer: 0.00005312
TECHNOLOGY TIP
Most calculators automatically switch to scientific
notation when they are showing large or small numbers that exceed the
display range. Try evaluating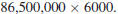 
If your
calculator follows
standard conventions, its display should be
which is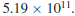 
Example 5 Using Scientific Notation with a Calculator
Use a calculator to evaluate
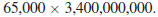
Solution
Because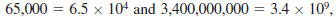 
you can
multiply
the two numbers using the following graphing calculator keystrokes.
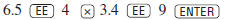
After entering these keystrokes, the calculator display
should read 
.
So, the product of the two numbers is
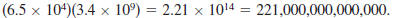
Now try Exercise 53.
Radicals and Their Properties
A square root of a number is one of its two equal factors.
For example, 5 is a
square root of 25 because 5 is one of the two equal factors of
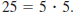In a
similar way, a cube root of a number is one of its three equal factors, as in
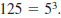
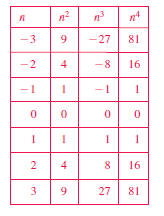
Use pattern recognition to help students
identify perfect squares, cubes, etc., of
both positive and negative integers when
simplifying radicals. Have students
construct a table of powers for several
integers. For example:
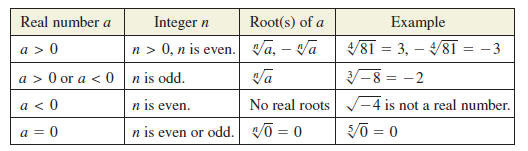
Definition of the nth Root of a Number
Let a and b be real numbers and let
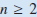be a positive integer. If
then b is an nth root of a. If n=2 the root is a square root. If n=3, the
root is a cube root.
Some numbers have more than one nth root. For example,
both 5 and -5
are square roots of 25. The principal square root of 25, written as
is
the
positive root, 5. The principal nth root of a number is defined as follows.
Principal nth Root of a Number
Let a be a real number that has at least one nth root. The
principal nth root
of a is the nth root that has the same sign as a. It is denoted by a radical
symbol
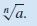Principal nth root
The positive integer n is the index of the radical, and
the number a is the
radicand. If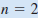 
omit the index and write
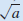rather than
(The
plural of index is indices.)
A common misunderstanding when taking square roots of real
numbers is
that the square root sign implies both negative and positive roots. This is not
correct. The square root sign implies only a positive root. When a negative root
is
needed, you must use the negative sign with the square root sign.
Example 6 Evaluating Expressions Involving Radicals
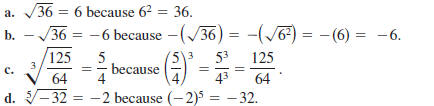
e.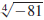 
is not a real
number because there is no real number that can be raised
to the fourth power to produce -81
Now try Exercise 59.
Here are some generalizations about the nth roots of a
real number.
Generalizations About nth Roots of Real Numbers
Integers such as 1, 4, 9, 16, 25, and 36 are called
perfect squares because
they have integer square roots. Similarly, integers such as 1, 8, 27, 64, and
125
are called perfect cubes because they have integer cube roots.
Properties of Radicals
Let a and b be real numbers, variables, or algebraic
expressions such that
the indicated roots are real numbers, and let m and n be positive integers.
| Property |
Example |
 |
 |
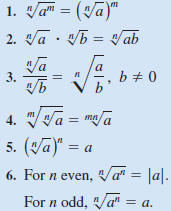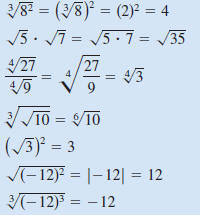Example 7 Using Properties of Radicals
Use the properties of radicals to simplify each
expression.
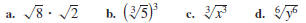
Solution
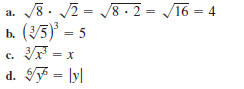
TECHNOLOGY TIP
There are three methods of evaluating
radicals on most graphing
calculators. For square roots, you
can use the square root key 
.
For cube roots, you can use the
cube root key 
(or menu
choice). For other roots, you can
use the xth root key 
(or menu
choice). For example, the screen
below shows you how to evaluate
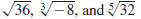using one
of the three methods described.
Additional Examples
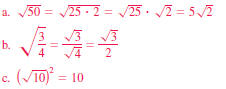
Now try Exercise 79.
Simplifying Radicals
An expression involving radicals is in simplest form when
the following
conditions are satisfied.
1. All possible factors have been removed from the
radical.
2. All fractions have radical-free denominators (accomplished by a process
called rationalizing the denominator).
3. The index of the radical is reduced.
To simplify a radical, factor the radicand into factors
whose exponents are
multiples of the index. The roots of these factors are written outside the
radical,
and the “leftover” factors make up the new radicand.
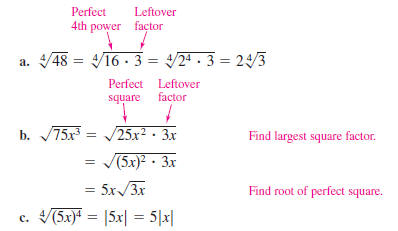
Example 8 Simplifying Even Roots
STUDY TIP
When you simplify a radical,
it is important that both
expressions are defined for the
same values of the variable.
For instance, in Example 8(b),
are both
defined only for nonnegative
values of x. Similarly, in
Example 8(c),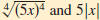
are both defined for all real
values of x.
Example 9 Simplifying Odd Roots
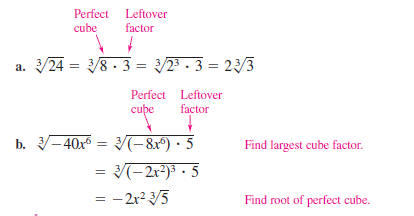
Now try Exercise 81(b).
Radical expressions can be combined (added or subtracted)
if they are like
radicals—that is, if they have the same index and radicand. For instance,
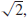
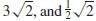are like radicals, but
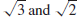are unlike radicals. To determine
whether two radicals can be combined, you should first simplify each radical.
Example 10 Combining Radicals
 |
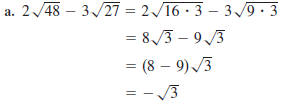Find square factors.
Find square roots and
multiply by coefficients.
Combine like terms. |
 |
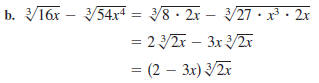Find cube factors.
Find cube roots.
Combine like terms. |
Now try Exercise 85.
Try using your calculator to check the result of Example
10(a). You should obtain
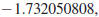which is the same as the calculator’s
approximation for 
Rationalizing Denominators and Numerators
To rationalize a denominator or numerator of the form
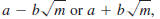
multiply both numerator and denominator by a conjugate:
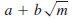and
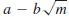are conjugates of each other. If a=0, then
the rationalizing factor
for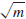 
is itself,
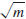. Note that the product of a number and its
conjugate is a
rational number.
Example 11 Rationalizing Denominators
Rationalize the denominator of each expression.
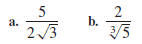
Solution
 |
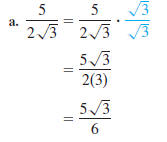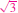 is
rationalizing factor.Multiply.
Simplify. |
 |
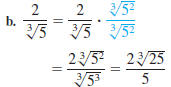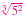 is
rationalizing factor.Multiply and simplify. |
STUDY TIP
Notice in Example 11(b) that
the numerator and denominator
are multiplied by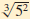 
to
produce a perfect cube radicand.
Example 12 Rationalizing a Denominator with Two Terms
Rationalize the denominator of
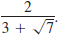
Solution
Additional Examples
Now try Exercise 93.
In calculus, sometimes it is necessary to rationalize the
numerator of an
expression.
Example 13 Rationalizing a Numerator
Rationalize the numerator of
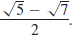
Solution
STUDY TIP
Do not confuse the expression
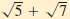with the expression
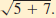In general,
does not equal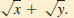 
Similarly,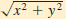 
does not
equal x+y.
Rationalizing the numerator is especially
useful when finding limits in calculus.
Now try Exercise 97.
Rational Exponents
If a is a real number and n is a positive integer such
that the principal nth
root of a exists, then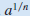 
is defined as
where 1/n is the
rational exponent of a.
Moreover, if m is a positive integer that has no common
factor with n, then
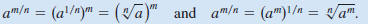
TECHNOLOGY TIP
Another method of evaluating
radicals on a graphing calculator
involves converting the radical to
exponential form and then using
the exponential key 
. Be sure
to use parentheses around the
rational exponent. For example,
the screen below shows you how
to evaluate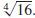 
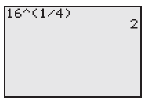
The numerator of a rational exponent denotes the power to
which the base is
raised, and the denominator denotes the index or the root to be taken.
When you are working with rational exponents, the
properties of integer
exponents still apply. For instance,
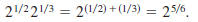
Example 14 Changing from Radical to Exponential Form
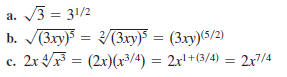
STUDY TIP
Rational exponents can be
tricky, and you must remember
that the expression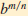
is not
defined unless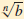 
is a real
number. This restriction produces
some unusual-looking
results. For instance, the number
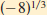is defined because
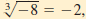but the number
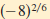is undefined because
is not a real number.
Additional Examples
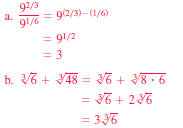
Now try Exercise 99.
Example 15 Changing from Exponential to Radical Form
Now try Exercise 101.
Rational exponents are useful for evaluating roots of
numbers on a calculator,
reducing the index of a radical, and simplifying calculus expressions.
Activities
Example 16 Simplifying with Rational Exponents
STUDY TIP
The expression in Example 16(e)
is not defined when x=1/2
because
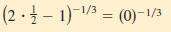
is not a real number.
Now try Exercise 107.
P.2 Exercises
Vocabulary Check
Fill in the blanks.
1. In the exponential form
is the _______ and is the _______ .
2. A convenient way of writing very large or very small numbers is called
_______ .
3. One of the two equal factors of a number is called a _______ of the number.
4. The _______ of a number is the th root that has the same sign as a and is
denoted by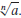 
5. In the radical form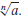 
the positive integer
is called the _______ of the radical and the number a is called the _______ .
6. When an expression involving radicals has all possible factors removed,
radical-free denominators, and a reduced index,
it is in _______.
7. The expressions 
are _______ of each
other.
8. The process used to create a radical-free denominator is known as _______ the
denominator.
9. In the expression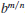 
, m denotes the _______
to which the base is raised and n denotes the _______ or root to be taken.
In Exercises 1–8, evaluate each expression.
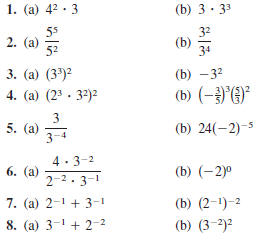
In Exercises 9–14, evaluate the expression for the
value of x.
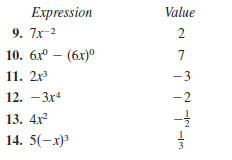
In Exercises 15–20, simplify each expression.
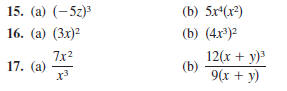
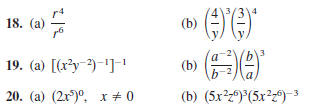
In Exercises 21–24, use a calculator to evaluate the
expression. (Round your answer to three decimal places.)
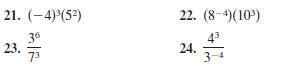
In Exercises 25–34, write the number in scientific
notation.
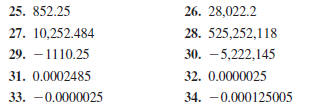
In Exercises 35–42, write the number in decimal
notation.
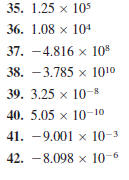
In Exercises 43–46, write the number in scientific
notation.
43. Land area of Earth: 57,300,000 square miles
44. Light year: 9,460,000,000,000 kilometers
45. Relative density of hydrogen: 0.0000899 gram per cubic
centimeter
46. One micron (millionth of a meter): 0.00003937 inch
In Exercises 47–50, write the number in decimal
notation.
47. Daily consumption of Coca-Cola products worldwide:
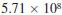drinks (Source: The Coca-Cola Company)
48. Interior temperature of sun: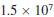 
degrees
Celsius
49. Charge of electron: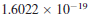 
coulomb
50. Width of human hai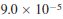r: 
meter
In Exercises 51 and 52, evaluate the expression without
using a calculator.
In Exercises 53–56, use a calculator to evaluate each
expression. (Round your answer to three decimal places.)
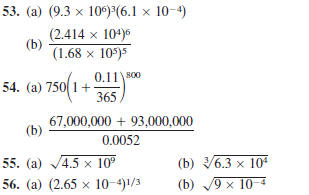
In Exercises 57– 66, evaluate the expression without
using a
calculator.
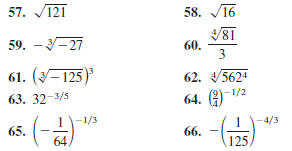
In Exercises 67–78, use a calculator to approximate the
value of the expression. (Round your answer to three
decimal places.)
In Exercises 79 and 80, use the properties of radicals
to
simplify each expression.
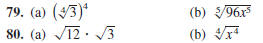
In Exercises 81–86, simplify each expression.
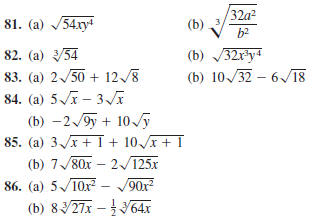
In Exercises 87–90, complete the statement with <, =,
or >.
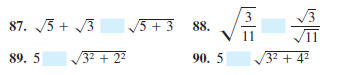
In Exercises 91–94, rationalize the denominator of the
expression. Then simplify your answer.
In Exercises 95–98, rationalize the numerator of the
expression. Then simplify your answer.
In Exercises 99–106, fill in the missing form of the
expression.
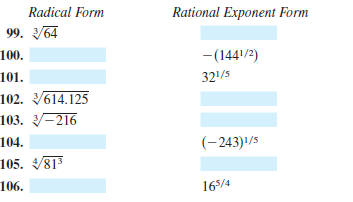
In Exercises 107–110, perform the operations and
simplify.
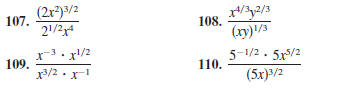
In Exercises 111 and 112, reduce the index of each
radical
and rewrite in radical form.
In Exercises 113 and 114, write each expression as a
single
radical. Then simplify your answer.
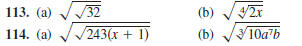
115. Erosion A stream of water moving at the rate of v
feet
per second can carry particles of size 
inches. Find
the size of the particle that can be carried by a stream
flowing at the rate of 3/4 foot per second.
116. Environment There was
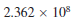tons of municipal
waste generated in 2003. Find the number of tons for each
of the categories in the graph. (Source: Franklin
Associates, a Division of ERG)
Section P.2 Exponents and Radicals
117. Tropical Storms The table shows the number of
Atlantic tropical storms and hurricanes per year from
1995 to 2005. Find the average number of tropical storms
and hurricanes from 1995 to 2005. Is your answer an
integer, a rational number, or an irrational number?
Explain. (Source: NOAA)
118. Mathematical Modeling A funnel is filled with
water to
a height of h centimeters. The formula
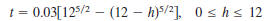
represents the amount of time t (in seconds) it will take
for
the funnel to empty. Find t for h=7 centimeters.
Synthesis
True or False? In Exercises 119 and 120, determine
whether the statement is true or false. Justify your answer.
121. Think About It Verify that
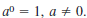(Hint: Use
the property of exponents 
122. Think About It Is the real number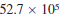 
written in
scientific notation? Explain.
123. Exploration List all possible digits that occur in the
units place of the square of a positive integer. Use that list
to determine whether 
is an integer.
124. Think About It Square the real number
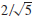and note
that the radical is eliminated from the denominator. Is this
equivalent to rationalizing the denominator? Why or why
not?
